2と、 1729と、 87539319と、・・・は何の数?
これらの数は、タクシー数(n番目のタクシー数をTa(n)と書く)というのですって!? 立方数の和に関する数であり、次のWikipedia を参照。
フリー百科事典『ウィキペディア(Wikipedia)』 タクシー数
https://ja.wikipedia.org/wiki/%E3%82%BF%E3%82%AF%E3%82%B7%E3%83%BC%E6%95%B0



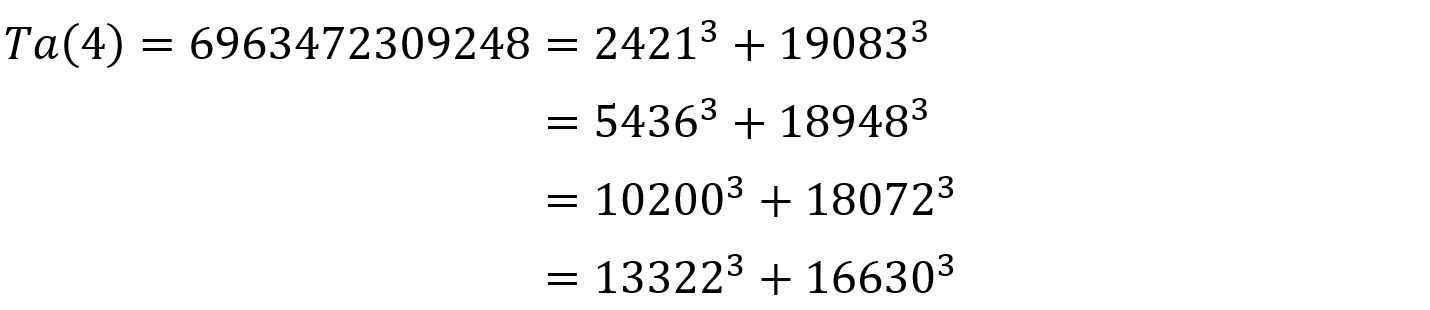
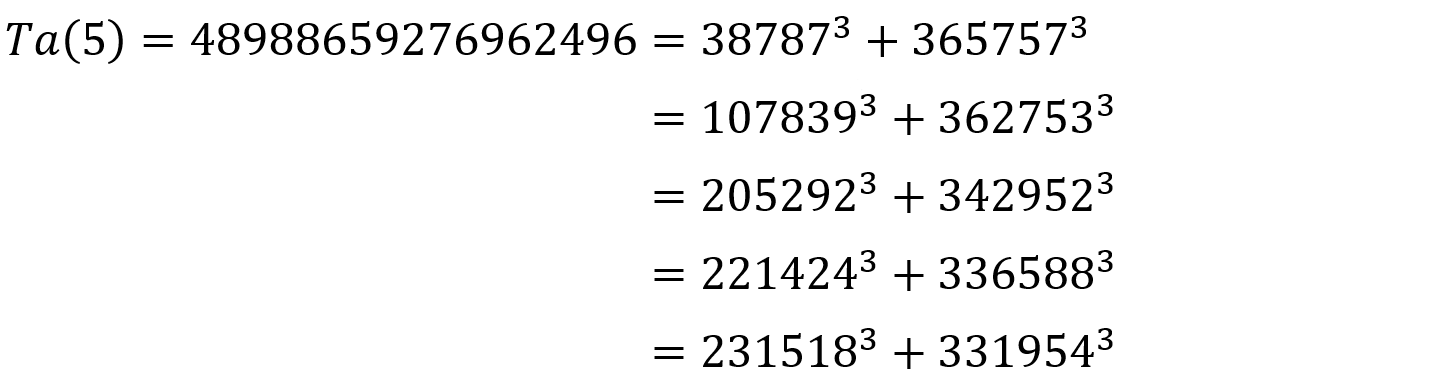

となり、現在まで上記のTa(6)までは、見つけられ証明されているけどTa(7)以上は、候補は見つけられているけど、未だ証明がされていないとか。ヨビノリさんの次のYouTubeで、わかりやすく説明してくれております。